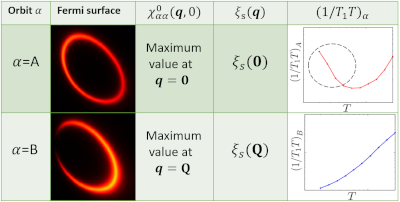
Motivated by recent nuclear magnetic resonance experiments, we calculated the spin susceptibility, Knight shift, and spin-lattice relaxation rate (1/T₁T) of the single-component molecular conductor [Ni(dmdt)₂] using the random phase approximation in a multi-orbital Hubbard model describing the Dirac nodal line electronic system in this compound. This Hubbard model is composed of three fragment orbitals and on-site repulsive interactions obtained using ab initio many-body perturbation theory calculations. We found fragment-orbital-dependent spin fluctuations with the momentum q=0 and an incommensurate value of the wave number q=Q at which a diagonal element of the spin susceptibility is maximum. The q=0 and Q responses become dominant at low and high temperatures, respectively, with the Fermi-pocket energy scale as the boundary. We show that 1/T₁T decreases with decreasing temperature, but starts to increase at low temperature owing to the q=0 spin fluctuations, while the Knight shift keeps monotonically decreasing. These properties are due to the intramolecular antiferromagnetic fluctuations caused by the characteristic wave functions of this Dirac nodal line system, which is described by an n-band (n≥3) model. We show that the fragment orbitals play important roles in the magnetic properties of [Ni(dmdt)₂].

(Click figure for a larger image.)